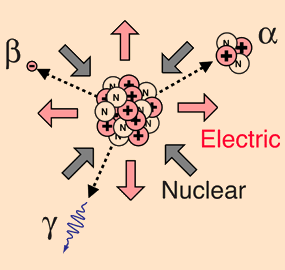
ELECTRON CAPTURE THROUGH BETA (β) RADIOACTIVITY

Beta Radioactivity

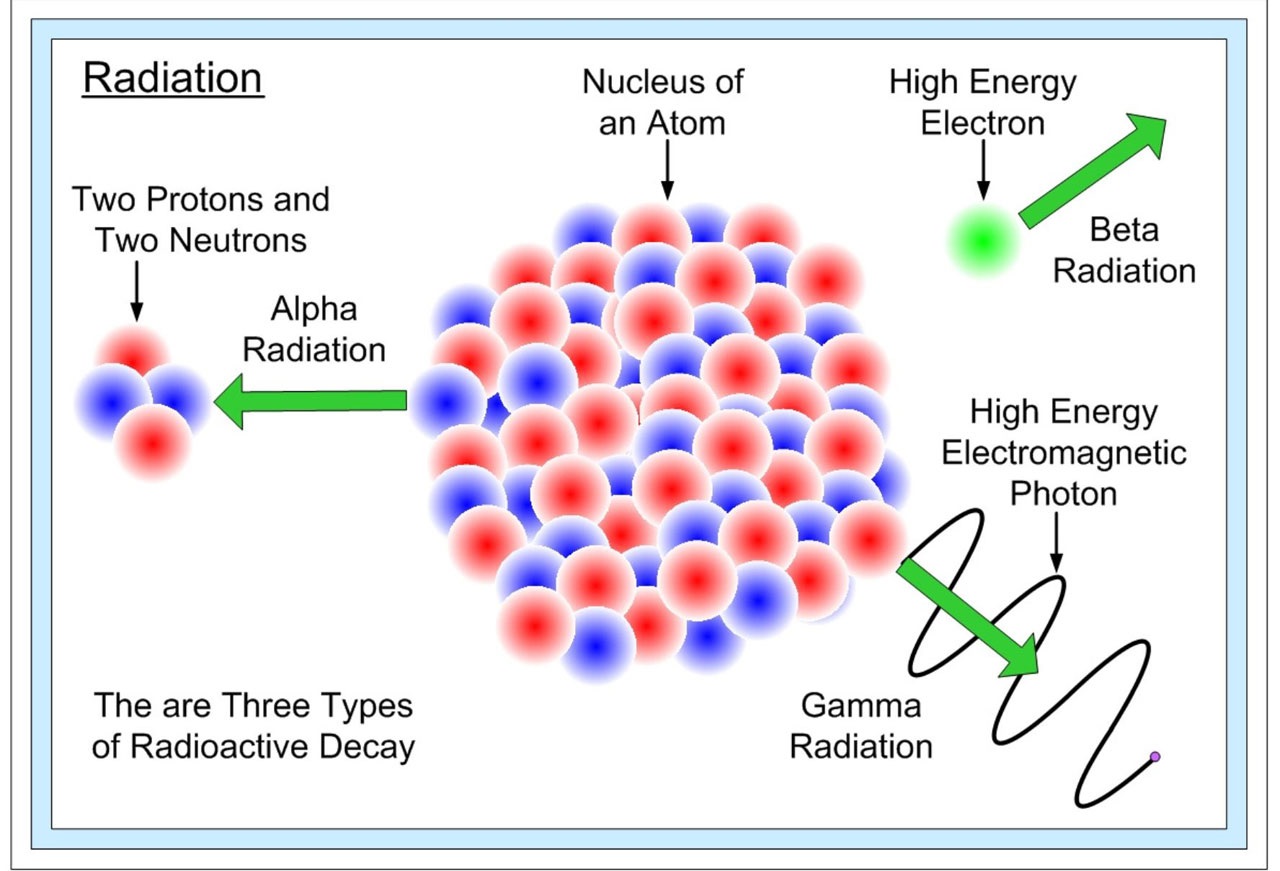
Image source: needpix.com
License: Royalty free , public domain

Beta decay occurs when, in a nucleus with too many protons or too many neutrons, one of the protons or neutrons is transformed into the other. In beta minus decay, a neutron decays into a proton, an electron, and an antineutrino: n Æ p + e - +. In beta plus decay, a proton decays into a neutron, a positron, and a neutrino: p Æ n + e+ +n. Both reactions occur because in different regions of the Chart of the Nuclides(see the below image), one or the other will move the product closer to the region of stability.

Image source:Wikimedia Commons
License: Creative Commons Attribution 3.0 Unported

These particular reactions take place because conservation laws are obeyed. Electric charge conservation requires that if an electrically neutral neutron becomes a positively charged proton, an electrically negative particle (in this case, an electron) must also be produced. Similarly, conservation of lepton number requires that if a neutron (lepton number = 0) decays into a proton (lepton number = 0) and an electron (lepton number = 1), a particle with a lepton number of -1 (in this case an antineutrino) must also be produced. The leptons emitted in beta decay did not exist in the nucleus before the decay–they are created at the instant of the decay.

An isolated proton, a hydrogen nucleus with or without an electron, does not decay. However within a nucleus, the beta decay process can change a proton to a neutron. An isolated neutron is unstable and will decay with a half-life of 10.5 minutes. A neutron in a nucleus will decay if a more stable nucleus results; the half-life of the decay depends on the isotope. If it leads to a more stable nucleus, a proton in a nucleus may capture an electron from the atom (electron capture), and change into a neutron and a neutrino.

Proton decay, neutron decay, and electron capture are three ways in which protons can be changed into neutrons or vice-versa; in each decay there is a change in the atomic number, so that the parent and daughter atoms are different elements. In all three processes, the number A of nucleons remains the same, while both proton number, Z, and neutron number, N, increase or decrease by 1.

In beta decay the change in binding energy appears as the mass energy and kinetic energy of the beta particle, the energy of the neutrino, and the kinetic energy of the recoiling daughter nucleus. The energy of an emitted beta particle from a particular decay can take on a range of values because the energy can be shared in many ways among the three particles while still obeying energy and momentum conservation.

Electron capture is concurrent to beta plus decay (i.e. in nuclei with too few neutrons). Instead of conversion of a proton into a neutron with a beta particle being emitted together with a neutrino, the proton captures an electron from the K shell: p + e --> n + ν.

The energy of the emitted beta particles is around 3 MeV, while their speed approximately corresponds to the speed of light.
Beta particles can penetrate matter. They lose energy in collisions with the atoms. There are actually two processes involved:

a beta particle transfers a small fraction of its energy to the struck atom

a beta particle is deflected from its original path by each collision and, since the change in the velocity leads to the emission of electromagnetic radiation, some of the energy is lost in the form of low-energy x-rays.

Notable radionuclides that undergo electron capture include ¹²³I, ⁶⁷Ga, ²⁰¹Th, and ¹¹¹In.
For example, ¹²³iodine undergoes electron capture to preduce ¹²³tellurium in excited state.

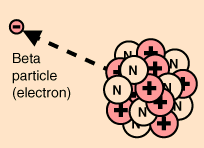
Beta particles are nothing but electrons from the nucleus. The high energy electrons have greater range of penetration than alpha particles, but still much less than gamma rays. The radiation hazard from betas is greatest if they are ingested.In the radioactivity process, the electrons are referred to as "beta" particle

Beta emission is accompanied by the emission of an electron antineutrino which shares the momentum and energy of the decay.

The emission of the electron's antiparticle, the positron, is also called beta decay. Beta decay can be seen as the decay of one of the neutrons to a proton via the weak interaction. The use of a weak interaction Feynman diagram can clarify the process.

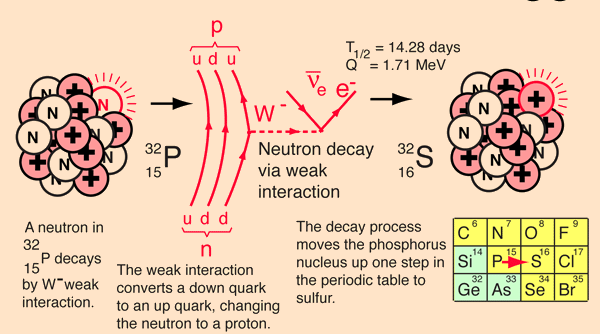
How Does The Beta Electron Escape All Those Protons?

Even though this is not plausible physically (e.g., the electron is not a point particle, it cannot be localized at such a point, etc.) it is an interesting conceptual question. An estimate of the electrical binding energy if it could be placed at the surface of the nucleus may be made by treating the ³²P nucleus as a sphere of charge and using the nuclear size model to calculate its radius. Then the electric potential energy could be calculated.

For a mass number A = 32 for ³²P , the nuclear size model yields r = 3.8 x 10^-15 m.
For ³²P with 15 protons, the nuclear charge Q = 2.40 x 10_-18-18 .

Using the radius and charge, the electric potential energy of the electron would be KQ_e/r = -9.09 x 10_-15 J = -5.68 MeV! It is hard to conceive of an electron escaping that!

Considering that placing an electron at the radius is not plausible, look at the entire beta decay process from the point of view of the nuclear binding energies involved. The process of beta decay must be examined from the point of view of the binding energy difference between the parent and daughter nucleus, and the energy yield is then distributed to the products of the decay.

The decay of ³²P can be considered to be a collective beta decay process, examined in more detail above. The total measured energy release Q is consistent with the identified nuclear processes. It can be calculated from the difference in neutral atomic masses for ³²P and ³²S.

The electron from the beta decay of ³²P can approach the full decay energy of 1.71 MeV, but it has an average value of about 0.7 MeV.
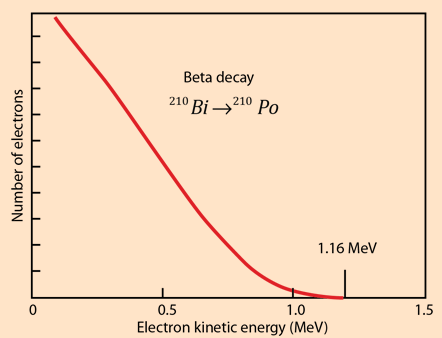
For another numerical example, consider the example of the beta decay of bismuth ²¹⁰Bi . For the beta decay ²¹⁰Bi -> ²¹⁰Po

Q_β- = [m(²¹⁰Bi) - m(²¹⁰Po)]c²

=(209.984095 u -209.982848 u)(931.502 MeV/u)

= 1.161 MeV

For beta decay, involving three particles, the beta electron energy is distributed from a low value up to the energy yield of the decay. The electron antineutrino carries most of the remaining energy, the recoil energy of the nucleus being very small.

Electron and Antineutrino

Early studies of beta decay revealed a continuous energy spectrum up to a maximum, unlike the predictable energy of alpha particles. Another anomaly was the fact that the nuclear recoil was not in the the direction opposite the momentum of the electron. The emission of another particle was a probable explanation of this behavior, but searches found no evidence of either mass or charge. The interesting history has Wolfgang Pauli in 1930 proposing an as yet unobserved particle to explain the continuous distribution of energy of the emitted electrons. Then Enrico Fermi called this particle a neutrino and developed a theory of beta decay in which the neutrino carried away the missing energy and momentum. With no charge and almost no mass, it was hard to detect, and not until 1956 was experimental detection of the neutrino achieved. For symmetry reasons, the particle emitted along with the electron from nuclei is called an antineutrino. The emission of a positron is accompanied by a neutrino.

Positron and Neutrino

The emission of a positron or an electron is referred to as beta decay. The positron is accompanied by a neutrino, an almost massless and chargeless particle. Positrons are emitted with the same kind of energy spectrum as electrons in negative beta decay because of the emission of the neutrino.

Beta Energy Spectrum

In the process of beta decay, either an electron or a positron is emitted. Because either a neutrino or an antineutrino is emitted as well, there is a spectrum of energies for the electron or positron, depending upon what fraction of the reaction energy Q is carried by the massive particle. The shape of this energy curve can be predicted from the Fermi theory of beta decay.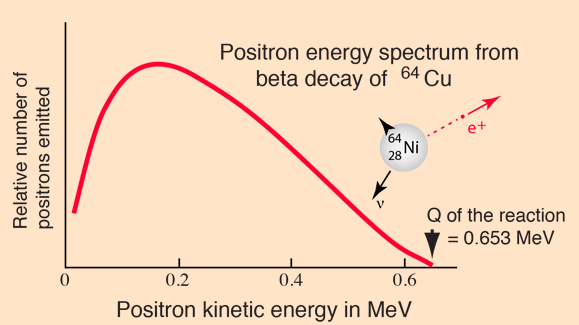

LIGHT ELECTRONS : A CHAOTIC JOURNEY THROUGH MATTER

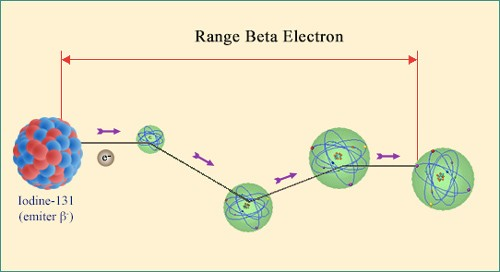
Trajectory of a beta electron:

The path of a beta electron emitted by a nucleus is long and chaotic. Like an alpha particle it is slowed down by ionisation but due to its tiny mass often undergoes deviations at large angles when it collides with heavy nuclei. Once most of an electron energy has been lost, it melts into the crowd of other electrons and eventually joins the existing atom cohort of orbiting electrons.

Beta electrons and positrons have equal and opposite electric charges. They are both emitted in the course of radioactive decays at speeds approaching that of light, and are therefore much faster than the heavier alpha particles.

A beta particle loses its energy (i.e slows down) by ripping electrons away from the atoms it passes: in other words, it ionises matter all along its trajectory. Hoxever, the path of an electron or positron differs dramatically from that of an alpha particle, whose mass is over 7300 times greater. A beta particle electron is indistinguishable from the electrons it interacts with.

When a beta particle and an atomic electron have a head-on collision, it becomes impossible to distinguish between the two particles and the energy is shared between the two miniscule, identical corpuscules. The nuclei approached also the paths of beta particles also help slow them down, by causing the emission of a gamma photon owing to their strong electromagnetic field (it is called a braking photon) which takes away a share of the beta particle energy.

When all is said and done, beta particles are less ionising than alpha particles, and their paths are random, long and sinuous. Beta radiation may be more penetrating, but its energy is deposited over a longer distance and is therefore less harmful in case of absorption. The differing levels of penetration can be shown in that alpha particles are stopped by human skin whereas beta particles can travel straight through. It takes up to three metres of air or seven millimetres of aluminium to stop the fastest of these particles.

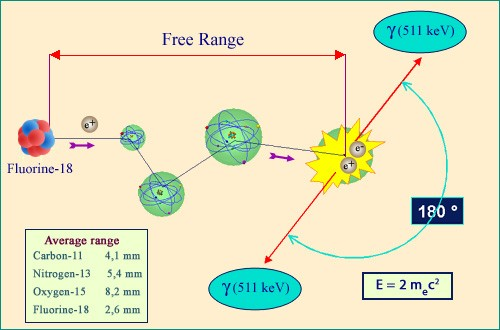
Birth, life and death of a positron

The trajectory of a positron emitted by a nucleus starts off in the same way as that of an electron. It is slowed by ionisation and deflected by collisions with heavy nuclei. Finally, however, when its speed is greatly reduced, a positron disappears in an annihilation collision with an atomic electron. Mutual antiparticles, the positron and the electron are entirely converted into energy. The released 1 MeV energy is carried away by two gamma photons, travelling in opposite directions.

The end of a positron path differs slightly from that of an electron, even though up to that stopping point their behaviours are almost identical. The difference is that when an electron loses all of its energy, it melts into a crowd of other, indistinguishable, electrons. A positron, however, will disappear.

This is because the positron, being a particle of antimatter, will find itself attracted to a electron. Pulled in by the opposite charges, the two particles will revolve around each other until they finally make contact. They will then mutually annihilate, releasing in the process almost a million electronvolts of energy which take the form of two gamma particles emitted back-to-back.

This classic signal of a positron disappearance has a wide range of medical applications, most famously in positron emission tomography, commonly known as PET.