Hydrozen Emission Spectral Lines & Derivation Of Rydberg Constant

Image credit: pixabay, Image Source

It is a well-known fact that when electrons in an atom or a molecule take in energy and become stimulated (or excited), they transit from a lower energy orbit to a higher energy orbit. And when they recoil back to the original lower energy state, they emit electromagnetic radiation in the form of photons. This physical process of absorption and emission of photons explains about the emission spectrum of Hydrozen.

The spectrum seen through a diffraction grating when a gas is stimulated by an electric discharge is made up of distinct, well-defined wavelengths rather than a continuous band of light. According to experiments, the wavelengths of the lines were typical of the chemical element that was generating the light. They were atomic fingerprints that came from the intrinsic structure of the atom.

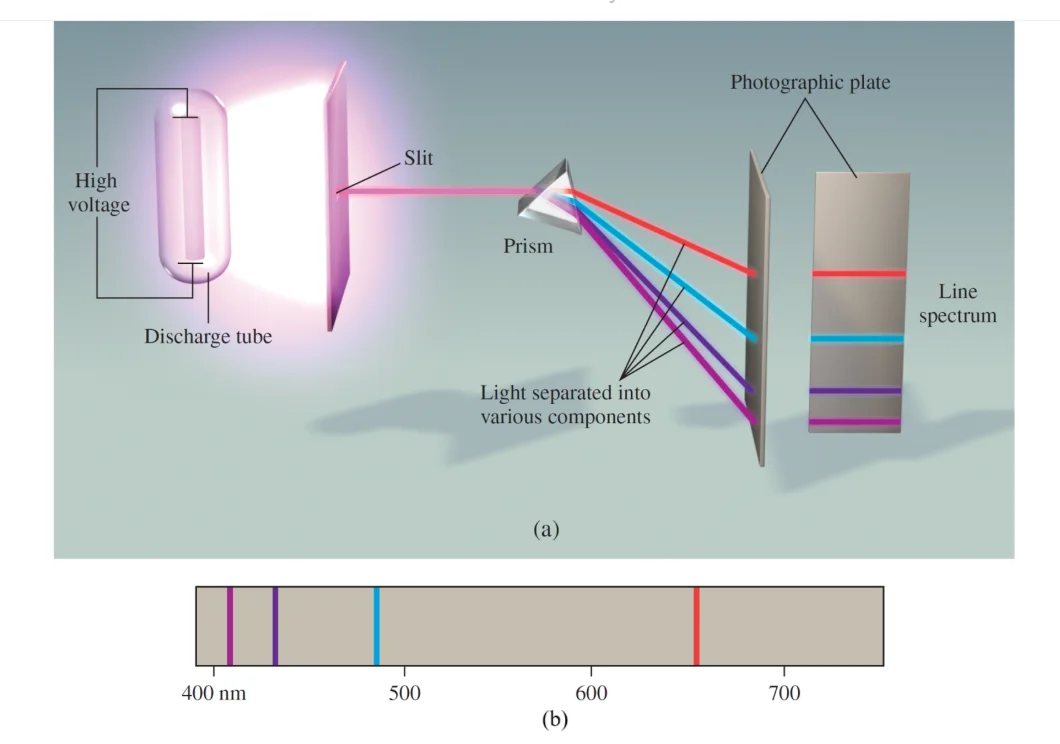
FIGURE-1: Line spectra of Hydrozen

An essential piece of evidence for demonstrating the quantized electronic structure of an atom is the hydrogen spectrum. When an electric discharge passes through a gaseous hydrogen molecule, the hydrogen atoms in the molecule dissociate. The highly excited hydrogen atoms cause electromagnetic radiation to be released as a result. The emission spectrum of hydrogen is made up of distinct frequency radiation. The scientists who made the discovery are honored by the names of these radiation series.

An electron undergoes a transition to a higher energy level when a photon is absorbed by a hydrogen atom, for instance, n = 1 n = 2. The electron changes from a higher energy level to a lower one when a photon passes through a hydrogen atom, for instance, n = 3 or n = 2. The transmission of light takes place during this change from one level to another. T he spectrum is made up of wavelengths that represent the variations in the quantized energy levels of the atoms. The line at 656 nm, for instance, represents the transition from n = 3 to n = 2.

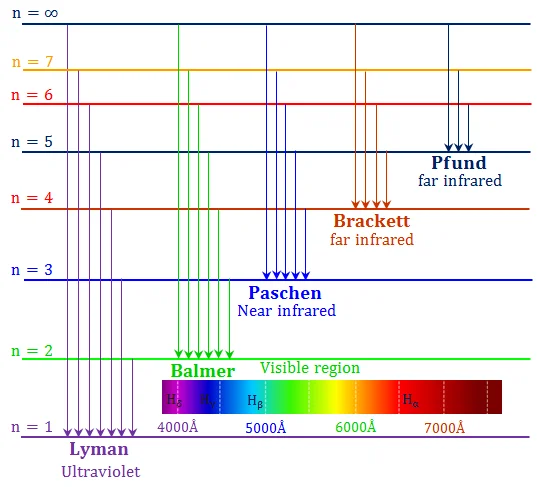
FIGURE-2: Hydrozen Spectrum Wavelengths

In experiments, it was found that hydrogen produced a variety of line series with distinct wavelength patterns. Throughout the spectrum, including the visible, ultraviolet, infrared, and other regions, the series repeated itself.

Researchers have found that the specific wavelength patterns of three such series - the Lyman, Balmer, and Paschen series - match. Despite the fact that each series' actual wavelengths vary, they all share the same traits: a large number of closely spaced wavelengths, followed by wavelengths that are farther apart, and finally some wavelengths that are quite distant.

The wavelengths of these series are provided by equations that experimenters have found. The following formulas describe how to obtain the wavelengths of this hydrogen series:

In all these series, R is the Rydberg constant, 10,973,731.6 m^-1, or about 1.097 x 10⁷ m^-1.

These equations suggest that atoms, especially hydrogen atoms, emit light only at certain wavelengths. This was because electrons couldn't simply continue to emit ever-smaller amounts of energy as only specific wavelengths were permitted.

Calculating The Shortest Wavelength Of The Balmer Series

What is the shortest wavelength in the Balmer series, the visible series? The formula for the Balmer series can be applied:

\( \cfrac{1}{λ} = R (\cfrac{1}{2^2} - \cfrac{1}{n^2}) \;\;\;\;\;\; n=3,4,5,... \)

The shortest wavelength (λ) corresponds to the biggest reciprocal \( 1 \over λ \) , and you get that when the \( 1 \over n^2 \) term goes to zero:

\( \cfrac{1}{n^2} → \;\; 0 \)
And that means that n → ∞. So for the shortest wavelength of the Balmer series, you have the following:


\( \cfrac{1}{λ} = R \cfrac{1}{2^2} \)

\( \cfrac{1}{λ} = R \cfrac{1.097 \times 10^7 m^{-1}}{2^2} \)

\( \cfrac{1}{λ} ≈ 2.74 \times 10^6 m^{-1} \)

The shortest wavelength in the Balmer series can be obtained by taking the reciprocal. What you get is as follows:

\( λ ≈ \cfrac{1}{2.74 \times 10^6 m^{-1}} ≈ 3.65 \times 10^{-7} m = 365 \; nm \)

Thus, 365 nm, or deep violet, is the shortest wavelength in the Balmer family.

Calculating The Longest Wavelength Of The Balmer Series

What about the longest wavelength of the Balmer series ? The formula for the Balmer series can be applied.

\( \cfrac{1}{λ} = R (\cfrac{1}{2^2} - \cfrac{1}{n^2}) \;\;\;\;\;\; n=3,4,5,... \)

When the 1/n² term is as large as possible, or when n = 3, you obtain the smallest reciprocal (1/λ), which is the longest wavelength (λ).

(That is the lowest value that the n in the Balmer series can have). Thus, for the longest wavelength of the Balmer series , you have
\( \cfrac{1}{λ} = R (\cfrac{1}{2^2} - \cfrac{1}{3^2}) \)

\( \cfrac{1}{λ} ≈ 1.52 \times 10^6 m^{-1} \)

The longest wavelength in the Balmer series can be obtained by taking the reciprocal.

\( λ ≈ \cfrac{1}{1.52 \times 10^6 m^{-1}} ≈ 6.56 \times 10^{-7} m = 656 \; nm \)

Additionally, 656 nm, or red, is the longest wavelength in the Balmer series. Thus, the majority of the visible spectrum, from deep violet to red, is neatly covered by the Balmer series.

Calculating The Rydberg Constant With The Hydrogen Line Spectrum

Considering Bohr's model of the hydrogen atom, the general formula for the wavelength at which electrons transfer in hydrogen is

\( \cfrac{1}{λ} = R (\cfrac{1}{n_{f}^2} - \cfrac{1}{n_{i}^2}) \;\;\;\;\;\; n_{f}=2,3,4,5,... , n_{i}=n_{f}+1, n_{f}+2, n_{f}+3,..... \)

where n_i is the initial energy level of the electron and n_f is the final energy level of the electron.

the wavelengths of the Balmer series of hydrogen is produced by
\( \cfrac{1}{λ} = R (\cfrac{1}{2^2} - \cfrac{1}{n^2}) \;\;\;\;\;\; n=2,3,4,5,... \)

That is because electron transitions from higher orbits to the n = 2 state are a part of the Balmer series.

The Lyman series is just the series with n_f = 1:

\( \cfrac{1}{λ} = R (\cfrac{1}{1^2} - \cfrac{1}{n_{i}^2}) \;\;\;\;\;\; n=2,3,4,5,... \)

The Paschen series is just the series with n_f = 3:

\( \cfrac{1}{λ} = R (\cfrac{1}{3^2} - \cfrac{1}{n_{i}^2}) \;\;\;\;\;\; n_{i}=4,5,6,... \)

Additionally, an electron in hydrogen atom has the following energy levels: see here for more details

\( E_{n} = \cfrac{-2π^2mk^2e^4}{h^2} \cfrac{Z^2}{n^2} \;\;\;\;\;\;\;\; n=1,2,3... \)

For photons, E = hf. And because \( f = \cfrac{c}{λ} \), that means that \( E = \cfrac{hc}{λ} \)

\( \cfrac{1}{λ} = \cfrac{E}{hc} \)

Thus, replace \( 1 \over λ \) in the equation with the value of E_n, and the result is

\( \cfrac{1}{λ} = \cfrac{-2π^2mk^2e^4}{h^3 c} \left( \cfrac{1}{n_{f}^2} - \cfrac{1}{n_{i}^2} \right) \;\;\;\;\;\;\;\; n_{f}=1,2,3... \;\;\; n_{i}=n_{f}+1,n_{f}+2,n_{f}+3... \)

Stated differently, according to the Bohr theory, the Rydberg constant, R, is equal to

\( R = \cfrac{-2π^2mk^2e^4}{h^3 c} \)

If we substitue the corresponding values in the above formula, R is calculated to be 10,973,731.6 m^-1, or about 1.097 x 10⁷ m^-1